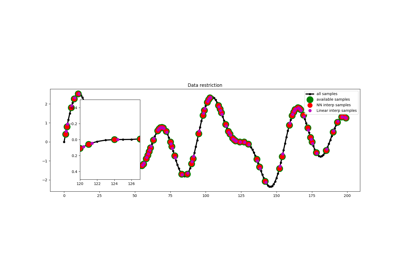
pylops.signalprocessing.Interp¶
-
pylops.signalprocessing.Interp(M, iava, dims=None, dir=0, kind='linear', dtype='float64')[source]¶ Interpolation operator.
Apply different kind of interpolations given a subset of values from input vector at locations
iava.Nearest neighbour interpolation is a thin wrapper around
pylops.Restrictionatnp.round(iava)locations.Linear interpolation extracts values from input vector at locations
np.floor(iava)andnp.floor(iava)+1and linearly combines them in forward mode, places weighted versions of the interpolated values at locationsnp.floor(iava)andnp.floor(iava)+1in an otherwise zero vector in adjoint mode.Note
the vector
iavashould contain unique values. If the same index is repeated twice an error will be raised. This also applies when values beyond the last element of the input array for linear interpolation as those values are forced to be just before this element.Parameters: - M :
int Number of samples in model.
- iava :
listornumpy.ndarray Floating indices of locations of available samples for interpolation.
- dims :
list Number of samples for each dimension (
Noneif only one dimension is available)- dir :
int, optional Direction along which restriction is applied.
- kind :
str, optional Kind of interpolation (
nearestandlinearare currently supported)- dtype :
str, optional Type of elements in input array.
Returns: - op :
pylops.LinearOperator Linear intepolation operator
- iava :
listornumpy.ndarray Corrected indices of locations of available samples (samples at
M-1or beyond are forced to be atM-1-eps)
Raises: - ValueError
If the vector
iavacontains repeated values.- NotImplementedError
If
kindis notnearestorlinear
See also
pylops.Restriction- Restriction operator
Notes
Linear interpolation of a subset of \(N\) values at locations
iavafrom an input (or model) vector \(\mathbf{x}\) of size \(M\) can be expressed as:\[y_i = (1-w_i) x_{l^{l}_i} + w_i x_{l^{r}_i} \quad \forall i=1,2,...,M\]where \(\mathbf{l^l}=[\lfloor l_1 \rfloor, \lfloor l_2 \rfloor,..., \lfloor l_M \rfloor]\) and \(\mathbf{l^r}=[\lfloor l_1 \rfloor +1, \lfloor l_2 \rfloor +1,..., \lfloor l_M \rfloor +1]\) are vectors containing the indeces of the original array at which samples are taken, and \(\mathbf{w}=[l_1 - \lfloor l_1 \rfloor, l_2 - \lfloor l_2 \rfloor, ..., l_M - \lfloor l_M \rfloor]\) are the linear interpolation weights.
This operator can be implemented by simply summing two
pylops.Restrictionoperators which are weighted usingpylops.basicoperators.Diagonaloperators.- M :