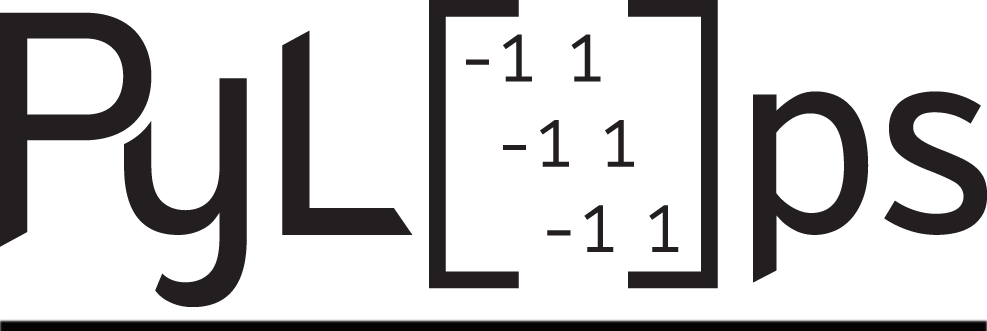Note
Go to the end to download the full example code
Identity#
This example shows how to use the pylops.Identity operator to transfer model
into data and viceversa.
import matplotlib.gridspec as pltgs
import matplotlib.pyplot as plt
import numpy as np
import pylops
plt.close("all")
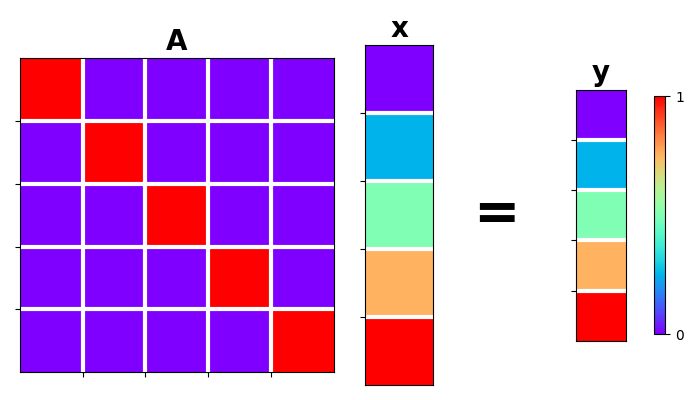
Let’s define an identity operator \(\mathbf{Iop}\) with same number of elements for data and model (\(N=M\)).
N, M = 5, 5
x = np.arange(M)
Iop = pylops.Identity(M, dtype="int")
y = Iop * x
xadj = Iop.H * y
gs = pltgs.GridSpec(1, 6)
fig = plt.figure(figsize=(7, 4))
ax = plt.subplot(gs[0, 0:3])
im = ax.imshow(np.eye(N), cmap="rainbow")
ax.set_title("A", size=20, fontweight="bold")
ax.set_xticks(np.arange(N - 1) + 0.5)
ax.set_yticks(np.arange(M - 1) + 0.5)
ax.grid(linewidth=3, color="white")
ax.xaxis.set_ticklabels([])
ax.yaxis.set_ticklabels([])
ax = plt.subplot(gs[0, 3])
ax.imshow(x[:, np.newaxis], cmap="rainbow")
ax.set_title("x", size=20, fontweight="bold")
ax.set_xticks([])
ax.set_yticks(np.arange(M - 1) + 0.5)
ax.grid(linewidth=3, color="white")
ax.xaxis.set_ticklabels([])
ax.yaxis.set_ticklabels([])
ax = plt.subplot(gs[0, 4])
ax.text(
0.35,
0.5,
"=",
horizontalalignment="center",
verticalalignment="center",
size=40,
fontweight="bold",
)
ax.axis("off")
ax = plt.subplot(gs[0, 5])
ax.imshow(y[:, np.newaxis], cmap="rainbow")
ax.set_title("y", size=20, fontweight="bold")
ax.set_xticks([])
ax.set_yticks(np.arange(N - 1) + 0.5)
ax.grid(linewidth=3, color="white")
ax.xaxis.set_ticklabels([])
ax.yaxis.set_ticklabels([])
fig.colorbar(im, ax=ax, ticks=[0, 1], pad=0.3, shrink=0.7)
plt.tight_layout()
Similarly we can consider the case with data bigger than model
x = [0 1 2 3 4]
I*x = [0 1 2 3 4 0 0 0 0 0]
I'*y = [0 1 2 3 4]
and model bigger than data
x = [0 1 2 3 4 5 6 7 8 9]
I*x = [0 1 2 3 4]
I'*y = [0 1 2 3 4 0 0 0 0 0]
Note that this operator can be useful in many real-life applications when for example we want to manipulate a subset of the model array and keep intact the rest of the array. For example:
\[\begin{split}\begin{bmatrix} \mathbf{A} \quad \mathbf{I} \end{bmatrix} \begin{bmatrix} \mathbf{x_1} \\ \mathbf{x_2} \end{bmatrix} = \mathbf{A} \mathbf{x_1} + \mathbf{x_2}\end{split}\]
Refer to the tutorial on Optimization for more details on this.
Total running time of the script: (0 minutes 0.249 seconds)